Mathematics has been a part of human culture for thousands of years, with its roots stretching back to ancient civilizations in Greece, Mesopotamia, and Egypt. Over the centuries, the greatest mathematicians have developed new theories and techniques that have transformed our understanding of the world around us. From Euclid’s geometry to Kepler’s laws of planetary motion, mathematics has been advanced by some brilliant minds who are remembered as some of the greatest mathematicians in history. In this article, I will explore their lives and contributions to mathematics so that we can gain an appreciation for how they shaped modern math.
Table of Contents
Why Are Mathematicians Important?
Mathematicians are important because they help us understand the world around us. Mathematicians think of new ways to solve problems and help us learn new things. In this article, we will learn about some people who were very smart at math and how they changed mathematics. We will discover what they did that was so special and why they are remembered as great mathematicians!
A Brief History of Mathematics: From Ancient Civilizations to Modern Times
Mathematics has been present in human societies since ancient times. One of the earliest civilizations to develop advanced mathematical systems was the Mesopotamians, who created a sexagesimal system with a base of 60. This system included mathematical concepts such as fractions, multiplication, and division. In Egypt, mathematics was used for practical purposes such as measuring land and building structures, resulting in the development of geometry.
The ancient Greeks are widely recognized as the foundation of modern mathematics, with philosophers such as Pythagoras and Euclid contributing significantly to its development. Pythagoras introduced his famous Pythagorean theorem for right-angled triangles, while Euclid laid down the foundations for geometry with his Elements, a systematic approach to mathematical proofs.
During the Middle Ages, mathematics continued to develop with the works of the greatest mathematician Al-Khwarizmi, who introduced the concept of algebra.
In the Renaissance, the Italian polymath Leonardo da Vinci incorporated mathematics into his work as an artist and inventor. Meanwhile, the greatest German mathematician Johannes Kepler developed his laws of planetary motion, which provided a mathematical explanation for the movements of planets around the sun.
The 17th century saw the emergence of one of the most influential figures in mathematics, Sir Isaac Newton. His work in calculus and physics revolutionized the field and continues to be an essential part of science.
In the 19th century, mathematics continued to progress with the work of mathematicians such as Carl Friedrich Gauss, who made significant contributions to fields such as number theory and geometry, and Augustin-Louis Cauchy, who developed the concept of limits in calculus.
The 20th century saw further advancements in mathematics with the development of new fields such as topology and logic, as well as groundbreaking work in areas such as probability theory and mathematical physics.
Today, mathematics continues to play a critical role in fields such as science, engineering, and finance, and mathematicians continue to make groundbreaking discoveries that shape our understanding of the world around us. Indeed, the history of mathematics is a testament to the enduring human curiosity and ingenuity that drives us to explore and unlock the mysteries of the universe.

The First Mathematician: Thales of Miletus
Thales of Miletus is widely considered to be the father of mathematics and one of the greatest pre-Socratic philosophers. Born in the ancient Greek city of Miletus in the 7th century BCE, Thales was a polymath who significantly contributed to mathematics, astronomy, and philosophy.
One of Thales’ most significant achievements was his discovery of the fundamental principles of geometry. He is credited with having laid the groundwork for the study of geometry with his discovery that certain geometric figures, such as the circle, have properties that are independent of the materials used to make them.
Thales also made key contributions to astronomy. He was one of the first to predict solar eclipses based on his understanding of the Earth’s rotation and the sun’s position in the sky. He is also said to have accurately predicted the size of the next harvest, using his knowledge of astronomical cycles and weather patterns.
Book recommendation: A History of Greek Mathematics, Vol. 1: From Thales to Euclid by Sir Thomas HeathIn addition to his achievements in mathematics and astronomy, Thales was also a philosopher who sought to understand the nature of reality. He believed that all things were made of water, an idea that was revolutionary for its time and contributed greatly to the development of ancient Greek philosophy.
Thales was a highly respected figure in ancient Greek society and was sought after as an advisor by kings and politicians. He founded a mathematical school in Miletus, and his teachings were passed down through his students, including Anaximander and Anaximenes, who would contribute to philosophy and science.
Overall, Thales’ contributions to mathematics and science laid the foundation for the great achievements of later mathematicians and scientists, such as Euclid, Pythagoras, and Isaac Newton. His legacy continues to be felt today, as his ideas and discoveries remain essential to our understanding of the natural world.
Euclid: The Father of Geometry

Euclid is highly regarded as one of the most influential figures in all mathematics. He was an ancient Greek mathematician and philosopher who developed a systematic approach to mathematical proofs, laying down the foundations for modern geometry with his book Elements.
His work focused on points, lines, angles, and parallelism and formulated many important ideas about circles, triangles, quadrilaterals, proportionality, and incommensurable magnitudes. Euclid’s work has been hugely influential throughout history and continues to be studied today as part of our understanding of geometry.
Euclid’s Elements is still considered one of the most influential mathematical works ever written and has been credited with revolutionizing mathematics. It contains some of the earliest known proofs, which have been studied and appreciated by generations of mathematicians, philosophers, and scientists.
Euclid is also credited with establishing a rigorous approach to logical reasoning, an essential part of mathematics today. His influence on Euclidean geometry and our modern understanding of mathematics cannot be overstated; he was one of the greatest minds in history.
Archimedes: The Father of Calculus

Archimedes is highly regarded as one of the most famous mathematicians and scientists of all time. Born in Syracuse, Sicily, in the 3rd century BCE, he made pioneering contributions to various fields, including mathematics, physics, engineering, and astronomy. Archimedes’ groundbreaking work in calculus is considered a cornerstone of modern mathematics.
One of Archimedes’ most significant mathematical achievements was the development of calculus, which he used to solve a variety of complex problems. Archimedes later created the “Method of Exhaustion,” which allowed him to calculate the area and volume of irregular shapes by partitioning them into smaller, more manageable pieces. He essentially invented the integral calculus method, which was later further developed by mathematicians such as Isaac Newton and Gottfried Wilhelm Leibniz.
Book recommendation: The Works of Archimedes In addition to his work on calculus, Archimedes made great contributions to the study of geometry, including developing the Archimedean Spiral and various formulas for calculating areas and volumes of geometric shapes.
Archimedes was also an important physicist and engineer known for his fluid dynamics and mechanics work. He is credited with discovering the principle of buoyancy, which states that the buoyant force acting on an object is equal to the weight of the displaced fluid. Archimedes is said to have made this discovery while taking a bath, leading to his famous exclamation of “Eureka!” (meaning “I have found it!”). This principle is still used today in a variety of applications, including the design of boats, submarines, and hot air balloons.
Reading recommendation: 12 Free Beautiful Calculus Lectures from 3Blue1BrownArchimedes was also an important inventor, having created a variety of machines and devices to help with engineering and warfare. He personally designed weapons and machines of war used by the armies of Syracuse during the Second Punic War, including the Archimedes Claw, a crane-like device used to topple ships, and the heat ray, which used mirrors to focus the sun’s rays onto enemy ships, setting them on fire.
Overall, Archimedes’ contributions to mathematics and science were revolutionary and continue to be studied and appreciated by mathematicians and scientists today. His work laid the foundations for many fields of study, including calculus, physics, and engineering. Archimedes is rightfully remembered as one of the greatest minds in the history of mathematics and science, and his legacy continues to inspire new generations of researchers and scholars.
The First Female Mathematician: Hypatia

Hypatia is considered to be the first female mathematician in history. Born in Alexandria, Egypt, in the 4th century CE, she was the daughter of Theon of Alexandria, another famous mathematician, and philosopher. Hypatia followed in her father’s footsteps and became a renowned mathematician and scholar in her own right.
Although much of Hypatia’s work has been lost to history, she is known to have made great contributions to the field of mathematics, particularly in the areas of algebra, geometry, and number theory. She was also an important teacher, sought out by students from all over the Mediterranean for her expertise.
Book recommendation: Hypatia: The Life and Legend of an Ancient PhilosopherHypatia was not only a talented mathematician but also a philosopher and scientist. She was well-versed in astronomy, mechanics, and philosophy and was known for her lectures and debates on these topics. Her teachings were open to all people, regardless of gender, race, or social status, and she was a strong advocate for education and intellectual freedom.
Hypatia’s life and work were cut short by her tragic death at the hands of a mob in 415 CE. Her murder, likely instigated by political and religious tensions in Alexandria then, was a devastating loss to the scholarly community. However, Hypatia’s legacy has lived on through the centuries, inspiring generations of women and mathematicians to pursue their passions and fight for equality and education.

Today, Hypatia is remembered as a groundbreaking figure in the history of mathematics and science, who defied societal expectations and achieved great intellectual heights. She paved the way for future generations of women in STEM fields, and her contributions continue to be studied and celebrated by scholars worldwide.
The Father of Algebra: Muhammad Al-Khwarizmi
Muhammad Al-Khwarizmi, born in Khwarezm, a region in modern-day Uzbekistan, during the 8th century, is widely acknowledged as the Father of Algebra. He was the greatest mathematician, astronomer, and geographer of his time.
In addition to his contributions to algebra, Al-Khwarizmi wrote extensively on diverse fields of mathematics, including spherical trigonometry, arithmetic, decimal fractions, and numerical methods. His work marked the beginning of the Golden Age of Islamic Science, a period of prolific advancements in various sciences and art forms.
Al-Khwarizmi’s greatest work, “Al-jabr wa Al-Muqabala,” which translates to “The Compendious Book on Calculation by Completion and Balancing,” led to the term “algebra” used today. In this book, he introduced algebraic symbols to represent unknown variables, such as x and y and provided a step-by-step method to solve mathematical equations. This innovation drastically changed how mathematics was viewed and approached, revolutionizing the field of algebra forever.
Book recommendation: Al-Khwarizmi: The Beginnings of Algebra by Roshdi RashidAl-Khwarizmi’s contribution to mathematics extended beyond the field of algebra. He also introduced the Arabic numeral system, a revolutionary development in mathematics that led to the creation of modern arithmetic. This system simplified arithmetic operations, which were previously difficult to perform using the Roman numeral system.
He was also an accomplished astronomer whose astronomical tables laid the foundation for future scientific discoveries in the field. His contributions significantly influenced the work of fellow mathematicians, such as the Italian mathematician Leonardo Fibonacci, who continued to advance mathematics in the following centuries.
Al-Khwarizmi’s work was crucial in transmitting knowledge from the East to the West, becoming a critical precursor to the European Renaissance. His legacy continues to inspire mathematicians today, as mathematical concepts developed him are still widely used in various fields of study, such as physics, engineering, and computer science.
In conclusion, Muhammad Al-Khwarizmi, the Father of Algebra, was an exceptional mathematician whose contributions to mathematics have shaped modern algebra and paved the way for numerous advancements in diverse fields of study. His work remains essential to the understanding and application of mathematical concepts, making him an invaluable figure in the history of mathematics.
The Most Talented Western Mathematician of the Middle Ages: Fibonacci

Fibonacci, also known as Leonardo of Pisa, was a great mathematician who lived in the Middle Ages and is recognized as one of the most talented in Western history. Born in Pisa, Italy, around 1170, Fibonacci traveled extensively throughout the Mediterranean world and became familiar with the mathematical systems of different cultures.
His most significant contribution to mathematics was introducing the Arabic numeral system to Western Europe. This numeral system, which uses Arabic numerals and a decimal point, revolutionized mathematical calculations, making them much simpler and more efficient. Previously, the Roman numeral system was used, which made calculations difficult and time-consuming.
Fibonacci’s book, “Liber Abaci,” meaning “Book of Calculation,” published in 1202, introduced the Arabic numeral system to the West. This book was instrumental in spreading the knowledge of this system and making it popular among merchants, bankers, and mathematicians. It also contained numerous mathematical problems and solutions, ranging from basic arithmetic to complex algebraic equations.
Book recommendation: Finding Fibonacci: The Quest to Rediscover the Forgotten Mathematical Genius Who Changed the World by Keith DevlinIn addition to introducing the Arabic numeral system, Fibonacci also discovered the Fibonacci sequence, a series of numbers where each number is the sum of the two preceding numbers. This sequence has many fascinating properties and is prevalent in nature, appearing in the growth patterns of plants and the shell structures of snails.
The Fibonacci sequence also connects to the “golden ratio,” a mathematical ratio of 1:1.618 often found in art and architecture. This ratio is believed to be aesthetically pleasing to the eye, and many artists and architects have incorporated it into their work.

Fibonacci’s work significantly impacted the development of mathematics and science in the Middle Ages and beyond. His contribution to the spread of the Hindu-Arabic numeral system revolutionized arithmetic and enabled mathematical calculations to be performed more efficiently. He also laid the foundations for modern algebra, inspiring future mathematicians to continue exploring and advancing these fields.
In conclusion, Fibonacci was a remarkably talented mathematician whose work profoundly impacted Western mathematics and science. His introduction of the Hindu-Arabic numeral system and the Fibonacci sequence laid the foundation for future developments in mathematics, and his legacy continues to inspire mathematicians to this day.
Johannes Kepler and His Laws of Planetary Motion

Johannes Kepler (1571-1630) was a great German mathematician, astronomer, and astrologer famously known for his laws of planetary motion. Kepler’s contributions to physics and astronomy significantly impacted our understanding of the cosmos and how planets move.
Kepler’s first law of planetary motion states that the planets move around the sun in elliptical orbits, with the sun at one of the ellipse foci. This law challenged the belief that planetary orbits were circular, a significant astronomical breakthrough. Kepler’s second law states that the line that connects a planet to the sun sweeps out equal areas at equal times, meaning that the planet moves faster when it is closer to the sun and slower when it is farther away.
This law helps to explain why planets move at varying speeds and why they seem to speed up or slow down at certain points in their orbit. Kepler’s third law states that the square of a planet’s orbit period is proportional to the cube of its average distance from the sun. This mathematical relationship describes the motion of planets in our solar system.
Kepler’s contribution to astronomy did not stop there. He also made significant progress in optics, leading to the modern telescope’s invention. In 1604, Kepler observed a supernova, a massive star explosion, and studied it in great detail using a telescope. He determined that this event was taking place outside our solar system and that there were parts of the universe beyond our starry sky.
Johannes Kepler’s findings and discoveries are highly regarded as significant in astronomy and physics. They helped pave the way for future advancements in these fields and allowed a more accurate understanding of our solar system and the cosmos. In recognition of his contributions, NASA’s Kepler mission, launched in 2009 to search for planets orbiting other stars, was named in his honor.
Kepler’s laws have been studied and taught in universities and astronomical societies worldwide and remain vital for researchers and enthusiasts interested in learning more about the universe. Overall, Kepler’s achievements have carved him a place in history as one of the most famous mathematicians and astronomers who ever lived.
The Father of Modern Science: Galileo Galilei

Galileo Galilei (1564-1642) was an Italian polymath often called the father of science. He was one of the most influential scientists! His contributions to physics, astronomy, and mathematics were groundbreaking and established him as one of the most important key figures in the scientific revolution.
Galileo’s work with celestial bodies led him to develop the telescope and use it for astronomical observations in the early 17th century. He was the first to observe the moons of Jupiter, the phases of Venus, and the rings of Saturn, which fundamentally changed our understanding of the solar system. Galileo’s observations also provided strong evidence for Copernican heliocentrism, the belief that the Earth and other planets revolve around the sun, which contradicted the Catholic Church’s geocentric views at the time.
Book recommendation: Dialogue Concerning the Two Chief World Systems: Ptolemaic and Copernican by Galileo GalileiIn addition to his work in astronomy, Galileo’s contributions to physics were also game-changing. He is credited with the modern concept of “inertia,” or the idea that an object will remain in motion or at rest unless acted upon by an external force. He also performed experiments on falling objects, which paved the way for the later development of calculus and Newton’s laws of motion.
However, Galileo’s ideas and observations did not come without controversy. He was brought before the Inquisition in 1633 and charged with heresy for supporting Copernican heliocentrism, which went against the Church’s teachings. Galileo was ultimately forced to recant his beliefs to avoid punishment, including imprisonment or death.
Reading recomendation: Galileo’s Drawings of the MoonDespite the censure he faced during his lifetime, Galileo’s scientific contributions are immeasurable. His work revolutionized our understanding of the natural world and laid the foundation for many scientific advancements, including the modern scientific method. Even today, Galileo’s legacy lives on as a symbol of curiosity, discovery, and the pursuit of scientific knowledge.
Gottfried W. Leibniz: The Last True Genius

Gottfried Wilhelm Leibniz (1646-1716), German’s greatest self-taught mathematician and philosopher, was one of the greatest polymaths in history. He is known as the last true genius due to his contributions to various fields, including mathematics, philosophy, law, and physics.
Leibniz is best known for his invention of calculus, considered one of the most important and revolutionary discoveries in the history of mathematics. He independently developed calculus simultaneously with Sir Isaac Newton, and the two men had a famous priority dispute. Despite this, Leibniz’s notations for differential equations have become the standard for calculus and are still used today.
Book recommendation: Leibniz on Binary: The Invention of Computer Arithmetic by Lloyd Strickland
In addition to calculus, Leibniz made major contributions to algebra and number theory. He developed a method for solving equations now known as linear differential equations, which are essential in many areas of physics and engineering. Leibniz also introduced the concept of binary arithmetic, which is the basis for modern computing.
Leibniz’s contributions were not limited to mathematics. He also made important contributions to philosophy, proposing the idea of monads, the basic constituent elements of the universe. Leibniz believed that everything in the universe was made up of monads and connected to form a unified whole. This idea had a significant influence on the development of modern philosophy.
Leibniz had a deep interest in law and politics, and he believed that these fields could benefit from the application of mathematical reasoning. He proposed a system for legal reasoning based on mathematical principles, which he called the “Mathesis Universalis”. This system aimed to create a universal language that would allow clearer and more precise communication in law and politics.
Despite his contributions to so many fields and his reputation as a polymath, Leibniz was never recognized in his lifetime as the true genius that he was. It was not until centuries later that his true value was fully appreciated. Today, Leibniz is remembered as one of the greatest minds in history and an inspiration to those who seek to understand the universe’s workings.
Isaac Newton: The Most Famous Mathematician of All Time
Isaac Newton is the most well-known and celebrated mathematician of all time. He is remembered not only for his groundbreaking work in mathematics but also for his contributions to physics, optics, and astronomy.
Born in 1642 in England, Newton was a highly gifted student with an early aptitude for science and mathematics. His work would eventually reshape our understanding of the universe and establish him as one of the greatest scientific minds in history.
Newton’s contributions to mathematics are vast and profound. He is credited with the co-invention of calculus, which he developed independently of Leibniz. Newton used calculus to develop a mathematical framework for understanding the physical world, laying the foundation for classical mechanics and allowing scientists to make accurate predictions about the behavior of moving objects.
Book recommendation: Isaac Newton by James GleickOne of Newton’s most famous mathematical works is his Philosophiæ Naturalis Principia Mathematica, commonly known as the Principia. In this groundbreaking text, Newton introduced his three laws of motion, which are still used today to explain the behavior of objects in motion.
In addition to his work in mathematics, Newton also made great contributions to the field of optics. He discovered that white light is made up of a spectrum of colors, and he invented the first reflecting telescope, which improved upon the limitations of the traditional refracting telescope.
Throughout his life, Newton maintained a deep interest in astronomy. He developed a theory of gravitation that explained the motions of the planets and other celestial bodies, later verified by observations and became known as Newton’s laws of gravitation.
Despite his many accomplishments, Newton was known for his reclusive personality and tendency to prioritize his work above all else. However, his contributions to science and mathematics have impacted our understanding of the natural world and continue to influence scientific inquiry.
In recognition of his work, Newton was knighted by Queen Anne in 1705 and served as President of the Royal Society from 1703 to 1727. He died in 1727 at 84, leaving behind a legacy that forever changed the course of scientific study.
Leonhard Euler: Lifelong Curiosity

Leonhard Euler is widely recognized as one of the greatest mathematicians ever. Born in Switzerland in 1707, Leonhard Euler demonstrated exceptional mathematical abilities from a young age. Throughout his life, he made countless contributions to a wide variety of fields, including infinitesimal calculus, graph theory, mechanics, and optics.
One of Euler’s most impressive accomplishments was his development of the “Euler’s formula.” This formula, often called the most beautiful equation in mathematics, relates five of the most important mathematical constants: e, i, π, 0, and 1. It has wide-ranging applications in fields such as electrical engineering and physics.
Book recommendation: Euler: The Master of Us Al by William W. DunhamLeonard Euler is also remembered for his work in calculus. In particular, he developed the concept of a function and created a unified notation system for calculus still in use today. This notation, which includes symbols such as f(x) and dy/dx, makes it easier to express complex mathematical ideas and has greatly contributed to the development of modern mathematics.
Another important contribution of Leonhard Euler was his work in graph theory. Euler is the founder of graph theory, which studies graphs and networks. Euler introduced the concepts of vertices, edges, and faces to the study of graphs. He developed the concept of the “Eulerian path” and “Eulerian circuit” to study the so-called “Seven Bridges of Königsberg” problem.

Leonhard Euler was also a prolific writer and produced a remarkable number of papers and books throughout his lifetime. In fact, it is estimated that he wrote over 800 papers and books, making him one of the most productive and greatest mathematicians in history.
Despite his many accomplishments, Euler experienced significant challenges in his life. He was born blind in his right eye and experienced vision problems. In addition, he suffered from several illnesses and underwent several surgeries that left him partially deaf. Nevertheless, he continued to work tirelessly throughout his life, displaying a lifelong curiosity and a deep passion for mathematics.
Leonhard Euler died in 1783 at the age of 76, leaving behind a legacy that continues to inspire mathematicians and scientists to this day. His impact on the field of mathematics cannot be overstated, and his contributions have forever changed how we think about and approach mathematical concepts. He is remembered as one of the greatest minds in history. His lifelong curiosity and dedication to mathematics continue to inspire those who seek to explore the mysteries of the universe.
Carl Friedrich Gauss: The Prince of Mathematics
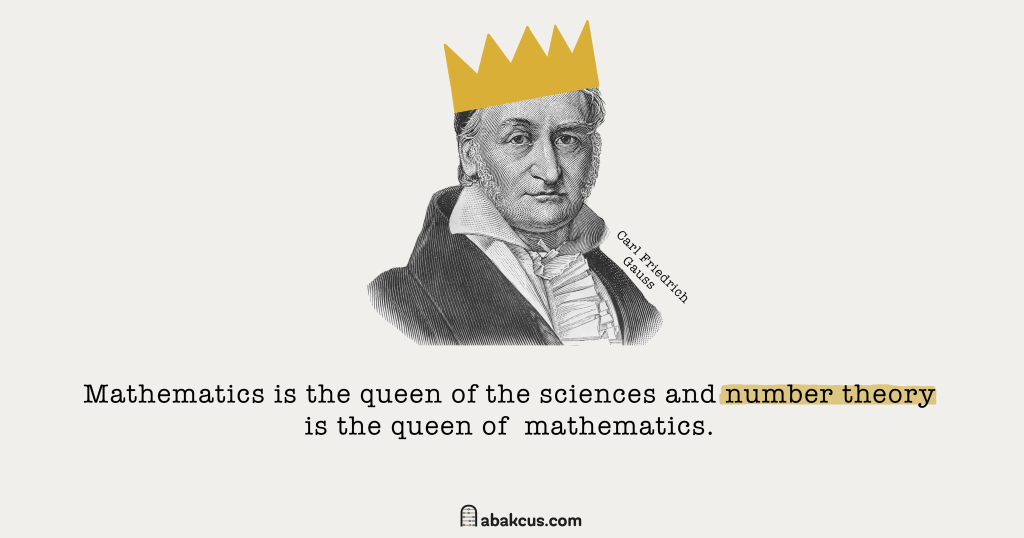
Carl Friedrich Gauss, also known as the Prince of Mathematics, was one of the finest mathematicians, physicists, and astronomers who made amazing contributions to a wide array of mathematical fields throughout his career. Gauss was born in Brunswick, Germany, in 1777, demonstrated exceptional mathematical abilities from a young age, and was considered a child prodigy.
Despite growing up in a relatively low-income family, Gauss quickly gained a reputation as a mathematical prodigy. At 18, he wrote a groundbreaking paper on the constructibility of regular polygons, considered a major foundational work in the field of algebraic geometry.
Throughout his career, Gauss made numerous groundbreaking contributions to mathematics, some of which are still considered among the most important discoveries in the field. He developed the least squares method, which is still used in various fields ranging from statistics to economics to modern physics. Gauss is also credited with discovering the fundamental theorem of algebra, which states that every non-constant polynomial equation has at least one complex root.
Book recommendation: The Prince of Mathematics: Carl Friedrich GaussGauss’s contributions to number theory were especially significant, and he developed the modular arithmetic theory, an important tool for cryptography. Gauss is also credited with discovering the law of quadratic reciprocity, which concerns the solvability of quadratic equations.
Gauss’s work in physics and astronomy was no less impressive. He is credited with the invention of the electric telegraph and worked extensively in the field of magnetism. In addition, Gauss made amazing contributions to the study of celestial mechanics, developing new methods for predicting the orbits of planets and discovering the asteroid Ceres.
Despite his many contributions to science and mathematics, Gauss was known for his extreme modesty and reluctance to publish his work. He famously stated that “mathematics is the queen of sciences and number theory is the queen of mathematics,” highlighting his deep respect for the field of mathematics.
Gauss died in 1855 at the age of 77, leaving behind a legacy that continues to inspire mathematicians and scientists to this day. His contributions to mathematics and science were immense, and his ideas were ahead of their time. His passion for mathematics and dedication to the pursuit of knowledge has left a lasting impact on the field of mathematics and continue to inspire young mathematicians worldwide.
Gauss is remembered as one of the greatest mathematicians of all time, and his legacy will continue to inspire generations of mathematicians to come.
Ada Lovelace: Founder of Scientific Computing

Ada Lovelace, also known as the Countess of Lovelace, was a pioneering mathematician and computer scientist who played a key role in developing early computing. Born in London in 1815, Lovelace was the daughter of Lord Byron, the famous poet, and his wife, Anne Isabella Milbanke, a wealthy mathematician and writer.
Lovelace’s mother provided her with a rigorous education in mathematics and science, believing that these subjects would help her daughter avoid the “insanity” that plagued her father. Lovelace quickly developed a passion for mathematics and went on to work with some of the most influential mathematicians of her time, including Charles Babbage, the inventor of the Analytical Engine, a design for a general-purpose mechanical computer.
Book recommendation: Ada Lovelace: The Making of a Computer Scientist by Christopher Hollings Lovelace’s work on the Analytical Engine was groundbreaking, as she realized that the machine could be programmed to perform a wide variety of functions beyond simple numerical calculations. Lovelace wrote in a famous letter to Babbage that the Engine “weaves algebraic patterns, just as the Jacquard loom weaves flowers and leaves.” Using a machine to perform symbolic operations is now recognized as the basis for modern computer programming.
In addition, Lovelace wrote the first algorithm intended to be processed by a machine. Her algorithm, designed for the Analytical Engine, was intended to compute the Bernoulli numbers, a sequence of rational numbers frequently arising in analytic number theory. Although the Analytical Engine was never completed, Lovelace’s work on it is now recognized as a major contribution to the history of computing and a precursor to modern computer science.
Lovelace’s legacy extends beyond her work on the Analytical Engine, however. She was a strong advocate for applying mathematics and science to the study of the natural world, and she wrote extensively on the topics of science and technology. She was also a champion of women’s rights and believed that women should have equal opportunities in education and work.
Despite her many contributions to mathematics and computing, Lovelace’s work was largely forgotten for years after she died in 1852. Not until the mid-20th century did her work be rediscovered and recognized for its importance. Today, Lovelace is known as a computer science pioneer and a symbol of the potential of women in technology.
In conclusion, Ada Lovelace was a groundbreaking pure mathematician and computer programmer whose work on the Analytical Engine set the stage for modern computers. Her contributions to the field of computing and her advocacy for women’s rights continue to inspire future generations of mathematicians, scientists, and engineers. Lovelace’s legacy is a testament to the power of imagination, curiosity, and dedication in pursuing knowledge.
Bernhard Riemann – The Notorius German Mathematician

Bernhard Riemann is known as one of the greatest mathematicians in history, with his groundbreaking work spanning the fields of mathematical analysis and geometry. Born in Bavaria, Germany, in 1826, Riemann had a humble upbringing and displayed a talent for mathematics from an early age.
Riemann’s academic career began at the University of Göttingen, where he studied under some of the most prominent mathematicians of his time, including Carl Friedrich Gauss. Under Gauss’s guidance, Riemann developed a deep interest in number theory, a field that would become one of his primary areas of research.
Riemann’s most famous contribution to mathematics is his work on the theory of functions of a complex variable. In particular, he developed the concept of the Riemann surface, a mathematical object used extensively in studying complex analysis. Riemann also contributed greatly to the study of differential equations and developed the concept of a Riemannian metric, an idea that forms the basis of modern differential geometry. His work is known as Riemannian geometry.
Book recommendation: Prime Obsession: Bernhard Riemann and the Greatest Unsolved Problem in Mathematics by John DerbyshireAnother area of research that Riemann is remembered for is the Riemann hypothesis, one of the most important unsolved problems in mathematics. The hypothesis, first proposed by Riemann in 1859, concerns the distribution of prime numbers and has far-reaching implications for number theory and cryptography. Over a century later, the Riemann hypothesis remains one of the most challenging problems in mathematics, with a million-dollar prize offered by the Clay Mathematics Institute for anyone who can solve it.

In addition to his groundbreaking work in mathematics, Riemann was also a gifted teacher and mentor. Many of his students went on to become leading mathematicians in their own right, and Riemann’s influence can still be felt in the work of contemporary mathematicians working in fields as diverse as string theory, algebraic geometry, and topology.
Despite his many accomplishments, Riemann’s life was cut tragically short. He died when he was 39 from tuberculosis, leaving behind a rich legacy of groundbreaking mathematical work that continues to inspire scholars and mathematicians today. Riemann’s profound contributions to mathematics have earned him a lasting place in history, and his insights have paved the way for countless advances in science, technology, and engineering. For instance, a special function in math called Riemann Zeta Function honors Riemann.
Georg Cantor: the Inventor of Set Theory

Georg Cantor is a name that is synonymous with the field of mathematics and, in particular, the study of sets. Born on March 3, 1845, in Saint Petersburg, Russia, Cantor’s work would revolutionize how mathematics was approached and has had a lasting impact on the field that continues to this day.
Cantor’s interest in mathematics began at an early age, and he went on to study at the University of Berlin under some of the most prominent mathematicians of the time. During this period, he developed his groundbreaking theory of sets, which would become the foundation for modern mathematics.
Central to Cantor’s set theory is the concept of infinity. Before Cantor, mathematicians and prominent scientists had largely ignored the concept of infinity, as it was seen as a notion that was too abstract and difficult to grasp. However, Cantor’s work challenged this assumption, and he set out to develop a rigorous theory of infinity.
Book recommendation: Georg Cantor: His Mathematics and Philosophy of the Infinite by Joseph Warren Dauben
One of Cantor’s most noteworthy contributions to the set theory was developing the concept of cardinality. This term refers to the size of a set, and Cantor discovered that there are different sizes of infinity, with some being larger than others. This concept formed the basis of his theory of transfinite numbers, which explored the idea of infinite quantities that exceeded the cardinality of the set of all natural numbers.

Cantor’s work was resisted by some mathematicians, who found his ideas too abstract and challenging to understand. However, his work became increasingly influential in the field of pure mathematics and has led to the development of many new branches of mathematics, including set theory, topology, and analysis.
Despite his great contributions to mathematics, Cantor was plagued by mental health issues throughout his life. He suffered from depression and anxiety, and his work was often met with criticism and rejection, which contributed to his struggles.
Despite these challenges, Cantor continued to develop his ideas, and his work profoundly impacted the field of pure mathematics. His insights into the nature of infinity, the size of sets, and the relationships between different mathematical concepts continue to inform contemporary research in mathematics. His legacy remains a powerful inspiration to new generations of mathematicians.
David Hilbert: the Most Influential Mathematicians of the 20th Century

David Hilbert is one of the most renowned mathematicians of the 20th century, known for his vast contributions to numerous fields, including geometric algebra, logic, applied mathematics, and number theory. Born on January 23, 1862, in Germany, David Hilbert’s work would significantly influence the development of modern mathematics, and his ideas continue to inspire and inform contemporary research across various fields.
Hilbert studied at the University of Königsberg and later became a professor at the University of Göttingen, where he began to develop his influential theories. One of Hilbert’s most notable contributions was his work on logic, which was critical in developing first-order logical calculus, also known as predicate calculus. This system allowed for the formalization of mathematical proofs, establishing a rigorous foundation for mathematics.
Book recommendation: Hilbert by Constance ReidHilbert’s work also extended to the field of number theory, where he developed the Hilbert class field theory, which provided a deeper understanding of the relationship between algebraic number fields and their Galois groups. Additionally, David Hilbert’s contributions to geometry led to the development of his axioms, which provided the foundation for modern geometric algebra.
Beyond his groundbreaking research, David Hilbert also significantly impacted the field of mathematics through his teaching and mentorship. Many of his students became leading mathematicians in their own right, and he provided crucial guidance and support at critical moments in their careers.
David Hilbert’s work was not without its critics, and his ideas were the subject of intense debate in the mathematical community during his lifetime. However, his influence on modern mathematics cannot be overstated, and his ideas continue to inform contemporary research across a range of fields.
Reading recommendation: The List of Hilbert’s Twenty-Three ProblemsIn recognition of his contributions, David Hilbert was awarded numerous honors throughout his lifetime, including the prestigious Sylvester Medal in 1908 and the Copley Medal in 1930. His legacy as one of the most brilliant and influential mathematicians of the 20th century remains firmly established, and his insights into the fundamental structures of mathematics continue to inspire new generations of scholars and mathematicians.
Emmy Noether: the Most Influential Female Mathematician

Emmy Noether is highly regarded as one of the most influential mathematicians of the 20th century and the most prominent female applied mathematician in history. Born in Germany in 1882, she faced numerous challenges in a male-dominated field. Still, her groundbreaking contributions to abstract algebra applied mathematics, and theoretical and mathematical physics would revolutionize how mathematicians and physicists approached complex problems.
Noether’s work focused on algebraic structures, and she developed a range of groundbreaking mathematical concepts that challenged existing theories about the nature of algebraic equations. Her most significant contribution was her formulation of Noether’s theorem, which provided a deep insight into the relationships between symmetries in physical systems and the underlying laws of nature.
Book recommendation: Emmy Noether's Wonderful Theorem by Dwight E. NeuenschwanderNoether’s work was highly influential in theoretical physics, and her concepts were critical in developing the theory of relativity and quantum mechanics. She also made important contributions to the study of algebra, topology, mathematical physics, and commutative algebra, and her ideas continue to inform contemporary research across various fields.
Despite her immense talent and groundbreaking contributions, Noether faced significant obstacles in her career, including discrimination and limited opportunities for women in academia. She was forced to work as an unpaid lecturer for many years, and only in her career was she able to secure a paid position at the University of Göttingen.
Despite these challenges, Noether remained committed to her work, and her legacy has profoundly impacted the field of mathematics. Her ideas continue to be studied and applied in a range of contexts, and she is remembered as a true pioneer in the field.
In recognition of her contributions, Noether was awarded numerous honors throughout her lifetime, including the prestigious Ackermann-Teubner Memorial Award in 1932. She was elected to the American Academy of Arts and Sciences in 1935. Today, she remains a powerful inspiration to young mathematicians and scientists worldwide, and her legacy continues to shape the way we think about complex mathematical problems.
Kurt Gödel: the Greatest Logicians in History

Kurt Gödel is known as one of the most brilliant logicians in history, whose work has profoundly impacted mathematics and beyond. Born in Austria-Hungary in 1906, Gödel displayed exceptional talent and quickly established himself as a leading figure in mathematical logic.
Gödel’s greatest contribution to mathematics is his incompleteness theorems, which demonstrated the inherent limitations of formal systems in mathematics. His work challenged the prevailing view that mathematical systems could be consistent and provable. Instead, it showed that any mathematical system powerful enough to express basic arithmetic truths would necessarily contain statements that were true but unprovable within the system itself.
Book recommendation: When Einstein Walked with Gödel: Excursions to the Edge of Thought by Jim HoltThis groundbreaking insight fundamentally changed the way mathematicians and logicians approached the foundations of mathematics and forced them to grapple with the concept of mathematical truth more nuancedly. Gödel’s work also had significant implications for philosophy and computer science, and his ideas played a crucial role in developing artificial intelligence and studying computational complexity.
Despite his immense contributions to the field, Gödel struggled with mental illness throughout his life and died at 71 after refusing to eat. He was known for his intense focus, meticulous attention to detail, and deep interest in philosophy and theology.
In recognition of his groundbreaking work, Gödel was awarded numerous honors throughout his lifetime, including the National Medal of Science in 1974 and the prestigious Albert Einstein Award in 1951. His legacy as one of the most brilliant and influential logicians ever remains firmly established, and his ideas continue to inspire and challenge mathematicians and philosophers worldwide.
Paul Erdős: the Most Productive Mathematician in History

Paul Erdős is highly regarded as the most productive mathematician in history, with an astounding 1,525 published papers throughout his career. Born in Hungary in 1913, Erdős displayed a prodigious talent for mathematics when he was a kid and published his first paper at 17.
Throughout his career, Erdős collaborated with hundreds of mathematicians from around the world, earning him a reputation as a “mathematical nomad.” He traveled extensively, attending conferences and visiting colleagues, and lived out of a suitcase for much of his life. His collaboration network was so extensive that mathematicians constructed an “Erdős number” to measure their distance from him regarding co-authorship.
Book recommendation: The Man Who Loved Only Numbers: The Story of Paul Erdos and the Search for Mathematical Truth by Paul HoffmanErdős was known for his unorthodox approach to problem-solving, often relying on insights and intuition rather than technical expertise. He was particularly interested in number theory and graph theory and made outstanding contributions in both fields. He was also known for his fascination with problems that could be explained to a non-mathematician in a few sentences and for his eccentricity, often wearing a beret and carrying a plastic bag filled with his belongings.
Despite his unconventional ways, Erdős was a highly respected mathematician, and his work profoundly impacted the field. He was particularly interested in problems related to Ramsey’s theory and made important contributions to the area. He also developed several important conjectures, many of which have yet to be proven.

Erdős was a deeply prolific mathematician, working tirelessly throughout his life to make discoveries and share them with the world. He was also a strong advocate for open collaboration, and the sharing of knowledge, and his legacy continues to inspire mathematicians and scientists around the world.
In recognition of his contributions, Erdős was awarded numerous honors throughout his lifetime, including the Wolf Prize in Mathematics in 1983 and the Leroy P. Steele Prize for lifetime achievement in Mathematics in 1985. He passed away in 1996, but his impact on the field of mathematics continues to be felt to this day. His work and dedication to the pursuit of knowledge serve as a reminder of the transformative power of human curiosity and collaboration.
Benoit Mandelbrot: the Pioneer of Fractal Geometry

Benoit Mandelbrot was a Polish-born French mathematician widely regarded as the pioneer of fractal geometry. He was born in 1924 in Warsaw and grew up in a family of Jewish intellectuals. Mandelbrot’s father was a talented musician, and his mother was a doctor.
Mandelbrot’s academic career began at the École Polytechnique in France, where he studied engineering. However, his passion for mathematics soon led him to switch to the study of pure math. After completing his doctorate in 1952, Mandelbrot began working as a research scientist at IBM, where he would continue to work for nearly forty years.
Mandelbrot’s interest in fractals began in the late 1960s, inspired by his work in computer graphics at IBM. He coined the term “fractal” to describe objects that exhibited self-similarity at different scales and began investigating the mathematical properties of these structures. Mandelbrot’s work in this area culminated in his book, “The Fractal Geometry of Nature,” published in 1982, which became a seminal work in the field.
Book recommendation: The Fractalist: Memoir of a Scientific Maverick by Benoit MandelbrotMandelbrot’s contributions to mathematics were significant and far-reaching. He developed a new branch of geometry, fractal geometry, which is related to chaos theory and has applications in various fields, from art to finance. His work has also been used to describe natural phenomena, such as coastlines, clouds, and mountains.
One of Mandelbrot’s most notable contributions to mathematics is the set that now bears his name, the Mandelbrot set. This set is generated by a simple mathematical formula that yields intricate, infinitely detailed patterns. The Mandelbrot set has become an icon of mathematical beauty and complexity and has captured the imagination of mathematicians and the general public alike.

Mandelbrot also had a deep interest in the practical applications of mathematics. He was particularly interested in the study of turbulence and developed a mathematical model of turbulence that has been used in fluid dynamics and other fields. His work on fractals has also been applied to image compression, which has revolutionized the field of digital imaging.
Mandelbrot was a prolific writer and lecturer, and his work inspired generations of mathematicians and scientists. He received numerous awards and honors throughout his lifetime, including the National Medal of Science in 1986 and the Japan Prize in 2003. He passed away in 2010, but his legacy as a pioneer in fractal geometry and a visionary mathematician continues to inspire and challenge mathematicians and scientists around the world.
Maryam Mirzakhani: the First Woman to Receive the Fields Medal

Maryam Mirzakhani was an Iranian mathematician who was the first woman to receive the Fields Medal, the most prestigious award in mathematics. She was born in Tehran in 1977 and showed early talent in mathematics.
Mirzakhani obtained her BSc in mathematics from the Sharif University of Technology in Tehran in 1999 and her Ph.D. from Harvard University in 2004 under the supervision of Curtis T. McMullen, a fellow Fields Medalist. After completing her Ph.D., she held research positions at the Clay Mathematics Institute, Princeton University, and Stanford University.
Mirzakhani’s research focused on the study of surfaces called Riemann surfaces, which are complex mathematical objects with applications in many areas of mathematics, including geometric topology, algebraic geometry, and complex analysis. She made remarkable contributions to understanding the moduli spaces of Riemann surfaces, which have numerous applications in physics and string theory.
Book recommendation: Maryam's Magic: The Story of Mathematician Maryam Mirzakhani by Megan ReidMirzakhani was awarded the Fields Medal in 2014 for her groundbreaking work in the field of dynamical systems and geometry of moduli space. The Fields Medal is often called the “Nobel Prize of mathematics” and is awarded every four years to mathematicians under 40 who have made crucial contributions to the field. Mirzakhani was the first woman to receive the award in its 80-year history.
In addition to her contributions to mathematics, Mirzakhani advocated for the rights of women and girls in science and mathematics. She was a role model for many young women around the world and worked to create opportunities and support networks for women pursuing careers in these fields.
Mirzakhani passed away in 2017 at the age of 40 due to complications from breast cancer. Her legacy as a brilliant mathematician, a trailblazer for women in science, and a humanitarian continue to inspire and influence mathematicians and scientists around the world. She is remembered for her unique approach to problem-solving, her insight and creativity, and her dedication to advancing the frontiers of mathematical knowledge.
John Horton Conway: the Inventor of Game of Life

John Horton Conway is a British mathematician who made notable contributions to a wide array of mathematical fields, including group theory, knot theory, coding theory, and number theory. However, he is best known for inventing the Game of Life, a simple cellular automaton that has captivated mathematicians, computer scientists, and enthusiasts since its creation in 1970.
Conway was born in Liverpool, England, in 1937 and showed an early aptitude for mathematics. He received his BA and MA degrees in mathematics from Gonville and Caius College, Cambridge University, where he also became a Fellow of the College. He then received his Ph.D. in number theory from the University of Cambridge in 1964.
Book recommendation: Genius At Play: The Curious Mind of John Horton Conway by Siobhan RobertsConway’s Game of Life is a set of simple rules that govern the evolution of cells on a two-dimensional grid. Each cell can be in one of two states, alive or dead, and the rules determine whether a cell will live, die, or become alive in the next generation based on how many of its neighbors are alive. Despite its simplicity, the Game of Life is computationally universal, meaning that any computation that a computer can perform can also be performed within the game’s rules.
The Game of Life has captured the imagination of mathematicians and computer scientists. It has been used to explore a wide range of mathematical and scientific concepts, from the behavior of populations of bacteria to the complexity of computation. It has also been used as a teaching tool to introduce students to concepts such as emergent behavior, self-organization, and complexity theory.
Conway was a prolific researcher and author, and his work has significantly impacted many areas of mathematics and science. He was awarded numerous honors throughout his lifetime, including the Berwick Prize of the London Mathematical Society, the Nemmers Prize in Mathematics, and the Kyoto Prize in Basic Sciences. He was also elected a Fellow of the Royal Society in 1981.
Reading recommendation: List of 23 Things Named After John Horton ConwayConway passed away in 2020 due to complications from COVID-19. His legacy as a brilliant mathematician, a pioneer in cellular automata, and a charismatic teacher continues to inspire and influence mathematicians, computer scientists, and enthusiasts around the world. His Game of Life is a testament to his creativity, curiosity, and passion for exploring the mysteries of the universe through mathematics.
Terence Tao: the Mozart of Mathematics

Terence Tao is widely regarded as one of the most accomplished mathematicians of the 21st century, with a career characterized by groundbreaking work and numerous awards and honors. Born in Adelaide, Australia, in 1975 to parents who were both medical doctors, Tao showed an early aptitude for mathematics and, by the age of two, was able to add and subtract single-digit numbers. He was a child prodigy like Carl Friedrich Gauss.
Tao’s talent was further developed by his parents, who encouraged him to explore mathematical puzzles and problems since he was a little kid. By the age of nine, he was taking college-level math courses. By 14, he had attained a perfect score on the International Mathematical Olympiad, the youngest person ever to do so.

Tao went on to study mathematics at Flinders University in South Australia, where he received his Bachelor’s degree at 16 and his Ph.D. from Princeton University at 21, under the supervision of the Fields Medalist Elias Stein. He then became the youngest professor in the history of the University of California, Los Angeles, at the age of 24.
Tao’s research has spanned a wide range of areas in mathematics, including number theory, analysis, and partial differential equations. He has contributed greatly to studying prime numbers and their distribution and has developed new techniques and methods for solving long-standing problems in number theory and analysis.
Among his many achievements, Tao is best known for his work on the Navier-Stokes equations, which describe the motion of fluids such as water or air. These equations are incredibly complex and difficult to solve, and until recently, no one has been able to prove that solutions to the equations exist for all time. In 2010, Tao announced a breakthrough in this area, showing that solutions to the Navier-Stokes equations exist for all time in a certain space.
Tao’s work won numerous awards and honors, including the Fields Medal in 2006, the Breakthrough Prize in Mathematics in 2015, and the Crafoord Prize in Mathematics in 2018. He is also a Fellow of the Royal Society, the National Academy of Sciences, and the American Mathematical Society.
Although he has achieved so much at a young age, Tao remains humble and focused on his work. In an interview, he said, “My philosophy is just to try to do things that are interesting and important and not worry too much about what everyone else is doing.”
Tao’s work has inspired numerous mathematicians and scientists around the world, and he is considered by many to be the “Mozart of mathematics” with a rare combination of technical skill and creative insight. His career has been characterized by a relentless pursuit of excellence and a deep love of mathematics. His contributions to the field will continue to inspire and challenge future mathematicians.
These mathematicians have changed our understanding of mathematics in many ways. They have contributed to developing new ideas, techniques, and theories that have revolutionized the field. Their work has helped shape our understanding of the world around us and has significantly impacted science and technology. Today, their discoveries continue to inspire and challenge mathematicians and scientists around the world.