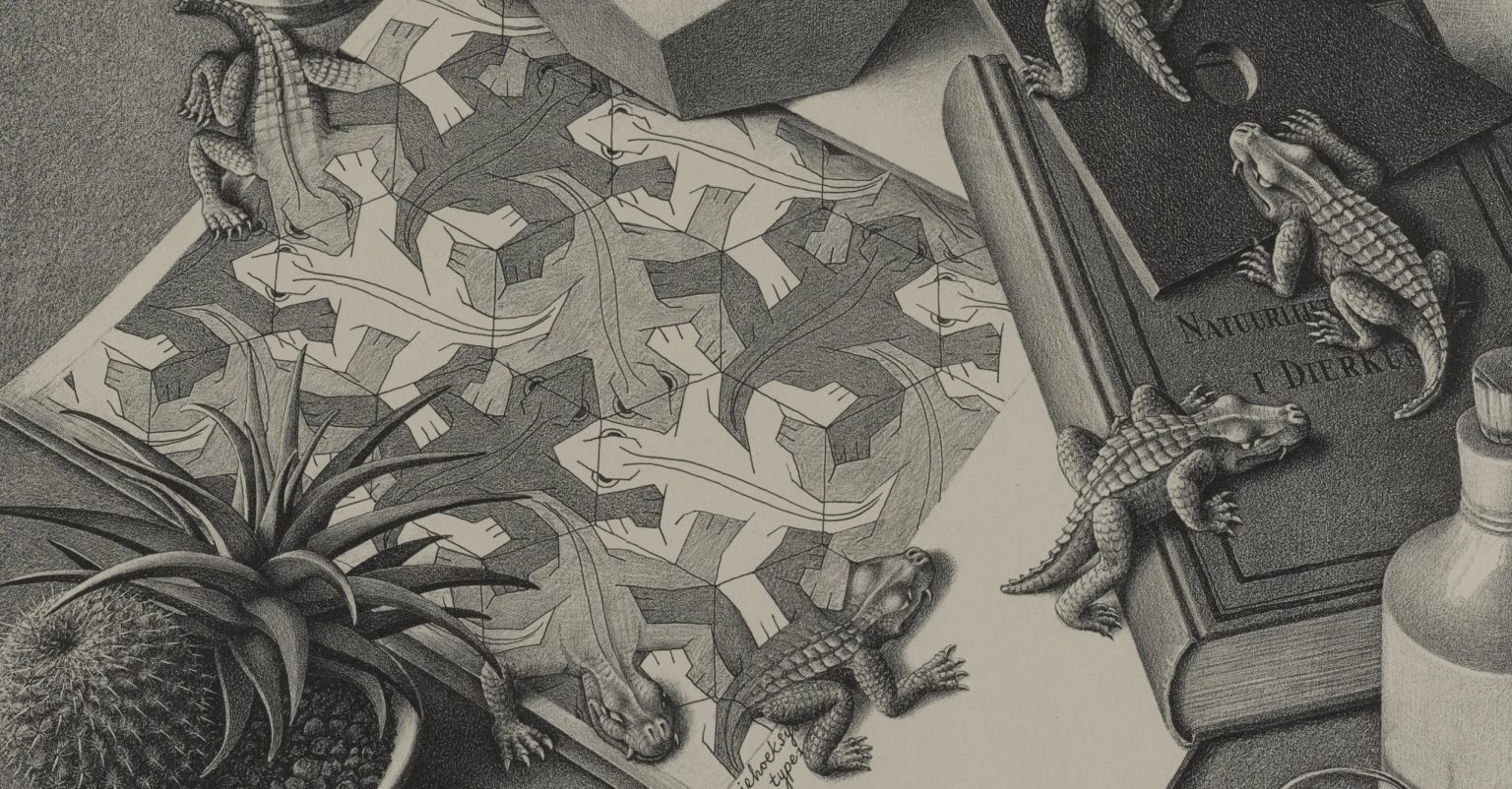
How does one describe the works of M.C. Escher? Brilliant? Mind-bending? Downright magical? Escher’s body of work is a rabbit hole of tessellations, impossible structures, and clever illusions that challenge the boundaries of reality as we know it. But among his many masterpieces, one piece sits at the whimsical, almost lizard-like intersection of art, mathematics, and a touch of surrealism. I’m talking about Escher’s Reptiles, a delightful lithograph from 1943 that demands a closer look—and trust me, it’s worth it.
Crawling from Paper to Reality
Imagine this scene for a moment: You’re looking at what seems to be the humble top of someone’s cluttered desk. There’s a sketchpad with a tessellation of little reptiles on it, their perfectly interlocking forms stretching across the page. However, instead of staying confined to the two-dimensional world, these reptilian shapes do something extraordinary—they come to life, emerging from the page as fully formed, three-dimensional creatures.
Yes, you read that correctly. These little guys climb right off the paper as if Escher had cast some kind of ancient wizardry. They crawl over the familiar objects scattered across the tabletop—a book, a set square, a cactus in a pot, and a rather prominent metal dodecahedron (because why not throw a 12-sided figure into the mix?). One adventurous reptile even lets out a puff of smoke atop that geometric pedestal—as you do when you’ve made it to the summit of “Desk Mountain.”
Eventually, their adventure ends where it began. They descend the tabletop and re-enter their tessellated grid, flattening back into two-dimensional linework. It’s like the circle of life—but for tessellated lizards.
The Genius of Escher’s Craft
Escher was many things—a mathematician, an artist, a man with a likely obsession for geometry—but above all else, he was a storyteller. What makes Escher’s Reptiles so mesmerizing isn’t just what’s depicted, but how he weaves motion, depth, and illusion into a static artwork.

1. Mathematics Meets the Magic of Tessellation
Escher’s fascination with tessellations—the seamless interlocking patterns that mathematicians dream of and puzzle enthusiasts adore—takes center stage in Reptiles. The reptiles themselves are built from geometrically precise outlines, packed together with no gaps or overlaps, like a lizard-based jigsaw puzzle.
What sets Reptiles apart is Escher’s evolution of these tessellated shapes. They begin as flat, abstracted forms but seamlessly transition into intricately detailed, textured creatures. The artist designs a gradient of detail and dimensionality, where these flat drawings morph into 3D beings. One moment, they’re just geometry—and the next, they’re climbing your desk as if auditioning for a role in Jurassic Park.
What can we say? The man knew his math.
2. The Visual Paradox
The scene itself feels rooted in reality—desk, cacti, books—familiar, mundane items that could sit on anyone’s workspace. But Escher adds a surreal twist. By juxtaposing such grounded objects with the absurdity of reptiles transcending their two-dimensional existence, he makes a delightful paradox. The reptiles exist in—but are also wildly out of—our reality.
The eye is immediately drawn to their cyclical motion, which is deliberate on Escher’s part. The composition’s circular flow encourages you to follow each lizard’s path, like tracking runners in a never-ending relay race.
3. Monochrome Madness
Escher’s choice to work in monochrome—restrained blacks, whites, and grays—is anything but dull. Think of it as a subtle flex. His meticulous shading and gradients make soft, rounded forms emerge from the page while giving textured surfaces (those lizard backs!) a level of realism that feels almost tangible.
This limited color palette also adds a moody layer to the scene, evoking an old, dream-like setting. You feel like maybe—just maybe—this surreal moment might actually happen if you stare hard enough at your own desk.
Bringing Mathematics to Life
Escher didn’t just draw lizards because he liked reptiles (although to be fair, who doesn’t?). For him, they were a means to explore deep mathematical concepts—symmetry, geometry, infinity. He fused these scientific principles into his art, making an entirely new visual language.
Take the dodecahedron in this piece, for example. It’s not just a randomly chosen object sitting there looking spiky and mysterious. It symbolizes the intersection of art and geometry, a nod to the mathematical precision Escher seamlessly integrated into his work. While others may have doodled in their notebooks during their math lectures, Escher emerged with tessellations—and a legacy.
A Dance Between Two Dimensions
If there’s one element that makes Escher’s Reptiles extraordinary, it’s the way Escher blurs the line between two-dimensional and three-dimensional space. Through this playful transformation, Escher challenges our perception of what’s possible. Escher’s reptiles aren’t just decorative motifs—they’re living, breathing characters in an infinite loop of emergence, exploration, and eventual return.
It’s a metaphor of sorts. Life itself is cyclical, full of moments where we rise (maybe even puff a little symbolic smoke) only to return once more to our starting point, grounding ourselves until the next adventure begins. Who knew a lithograph from 1943 could inspire some existential pondering?
Why Reptiles Still Fascinates Us Today
Decades later, Escher’s Reptiles continues to enchant viewers because it’s more than a piece of art—it’s an experience. It invites you to engage in the act of discovery, finding meaning in every scaled back and dodecahedral surface.
Escher’s ability to combine everyday objects and complex mathematical ideals into something playful yet profound makes Reptiles a timeless masterpiece. And the lizards themselves? Well, they serve as a reminder that sometimes, the most extraordinary things emerge from the simplest forms on paper.
Escher’s Call to Adventure
Perhaps the greatest gift of Escher’s art is this—it encourages us to look beyond the obvious, to play with the boundaries of possibility and reality. His lizards don’t just crawl across his desk—they crawl into our imaginations, daring us to think, “What else could happen, if only we dared to reimagine the rules?”
It’s an idea worth dwelling on. A little tessellation might just go a long way.