I’ve curated a stunning collection of 100 Google Earth images from the illustrious book Overview for your viewing pleasure! …
These books demystify complex concepts, illustrate the application of math in various fields, and share stories of mathematical discovery that inspire awe and wonder….
The 20+ history of science books listed below promise more than just an academic foray into the chronicles of scientific inquiry; they serve as a testament to the nobility of…
In this compilation, I present the top 10 Islamic geometry books, each offering a unique perspective on this captivating subject….
The books about bees listed here are but a few samples of the delightful troves of bee literature that await the curious reader. As you turn the pages of these…
This list of books about application of mathematics illuminates the diverse applications of mathematics, showcasing how this abstract science shapes our reality, influences various fields of research, and addresses complex…
In this guide, I delve into the best 5 graphing calculators that stand out in their field, helping engineers and students alike to achieve their academic and professional goals effectively….
Below I’ve curated a list of the seven best artificial intelligence books, perfect for those keen to learn about the intriguing world of AI, its capabilities, mysteries, and its potential…
A love for science often begins with a captivating book and the ones listed here have the power to ignite that spark. These science books are not only the highest-rated…
Help us exist with
Patreon
Get Abakcus straight to your inbox
Featured Directories

100 Breathtaking Google Earth Images from Overview
I've curated a stunning collection of 100 Google Earth images from the illustrious book Overview for your viewing pleasure!

100 Beautiful Math Poster Designs for Your Classroom
Are you looking for math poster ideas for your classroom? Then you'll love my poster designs! Math posters are a great way to learn about and explore the world of…
Best Products
When it comes to buying any kind of tech, it’s easy to pick the wrong thing. That’s where our experts and testing team come in to help guide your buying decisions.
Watch

Toposcape
An audiovisual piece made up of over four thousand still images pulled from Google Earth, individually edited, and then manually sequenced to Midnight by Caravan Palace.

Braving the Chill: -37F Winter in Yellowstone National Park
Winter in Yellowstone transforms into a land of extremes: the collision of boiling water from the park’s famed geysers with the Frost King’s touch creates an ethereal tableau of steam…
- Nature ,

The Physics of Kung Fu
In an intersection of martial arts and digital artistry, Tobias Gremmler, a German visual artist, captures the spirit of Kung Fu through his captivating digital renderings.
Tools

Literature Clock
The Literary Clock depicts the current time in the form of literary quotations and serves as an example of material and architectural design.
Steve Jobs Archive
The Steve Jobs Archive provides individuals with the resources and opportunity necessary to make their own contribution while simultaneously maintaining a reverence for the past and an excitement for the…
Featured Books
Listen

Stuff To Blow Your Mind
Stuff To Blow Your Mind is a beautiful podcast about neurological quandaries, cosmic mysteries, evolutionary marvels and our future.
- Science ,

Radiolab
For those looking for a science podcast that isn't afraid to get creative, Radiolab is the perfect pick. This show has long been hailed as the grandfather of science podcasting,…

Purple Rocket Podcast
Purple Rocket Podcast is a beautiful award-winning children's podcast that is full of excitement and adventure!
- Children ,
DIY
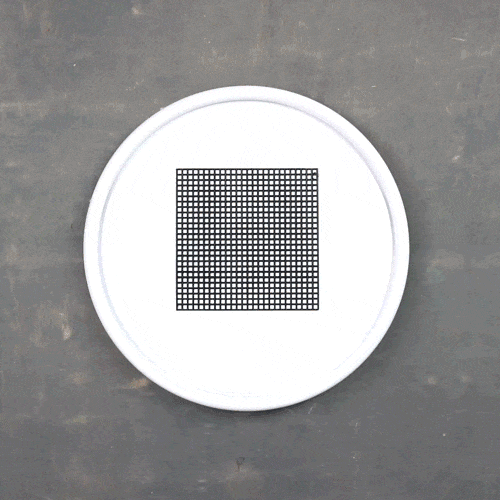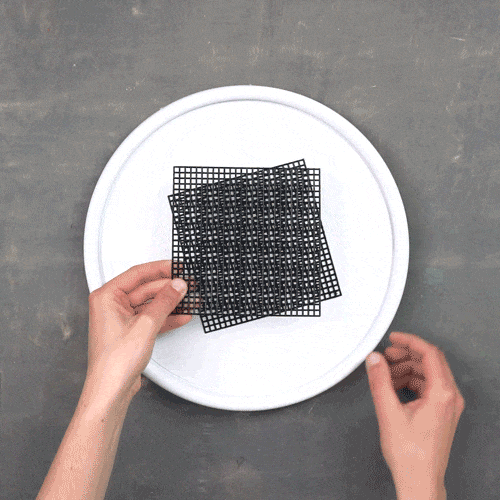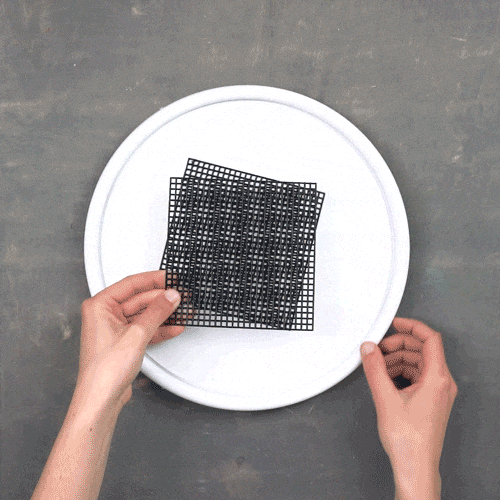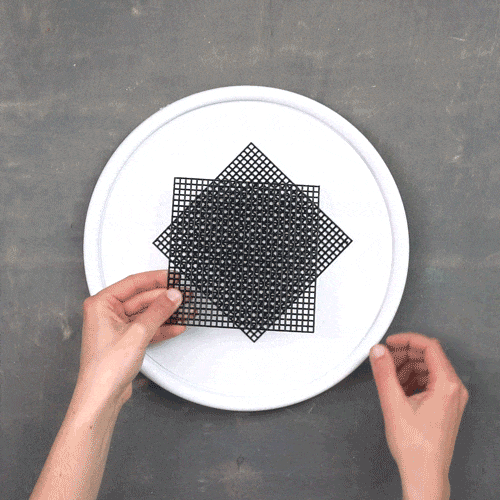
How to Make Moiré Patterns?
Have you ever looked through an electric fan and seen a shimmering pattern? Here is a beautiful math projects show that happens.

How to Make a Weather Balloon?
Make a barometer and predict the weather. As the air is sealed inside the jar, any changes to the air pressure outside the jar will direct the movement of the…

Scared Pepper Science Experiment
Move pepper away from you without touching it. Water bulges typically up a bit. You can see this by looking at a raindrop or filling a glass slightly over the…


















